A Key to Effective Instruction
by Kathy Richardson
As children develop an understanding of number concepts, there are certain essential ideas that are milestones, or hurdles in their growth of understanding. I have identified these stages of learning as Critical Learning Phases. According to the dictionary, a phase is “A particular moment or stage in a process, especially one at which a significant change or development occurs or a particular condition is reached.” The Critical Learning Phase that a child has reached determines what kinds of instruction will be meaningful and effective. Instruction which is given before a child has the foundational ideas necessary to understand the mathematics is not only ineffective but often leads to misconceptions.
In order to provide the most effective instruction, teachers need to understand how children develop an understanding of number concepts. Children generally move from thinking of numbers as a collection of single units (that is, one and another one and another one) -to seeing that one number is contained within another number- to learning the parts of numbers to 6 and then to 10. The next big milestone is recognizing that numbers are composed of tens and ones and eventually understanding numbers as hundreds, tens and ones.
At every stage of development, the size of the numbers and the size of the difference between numbers influences what the child is able to understand. That is, a child may be able to see that 5 is contained in 7 but not yet know how 15 is related to 17. Or a child may know the parts of numbers to 4 but not be able to figure out the parts of 6.
The level of abstractness also effects what children are able to think about. As children develop their understanding of number, they begin with models, moving them to aid their thinking. They generally advance from being able to think about numbers when they can actually move objects -to thinking about relationships when the model is present but not touched- to thinking about relationships without a physical model. Only after children can work with an idea at all these levels will their work with the symbolic representations of this idea have meaning for them. Even then, young children rarely reach the level of thinking that is totally abstract. When working with symbols they usually need to be thinking of some concrete referent.
Each stage of learning is much more complex than is generally recognized. If we look at counting for example, we will see that children who are competent counters have integrated several major ideas about counting. These include one to one correspondence, inclusion, keeping track, remembering how many, and knowing one more and one less. A child who understands counting will be able to keep track of an unorganized group of objects and will also be able to count out a particular quantity from a larger group of objects.
The following shows the stages children move through on their way to reaching just one aspect of counting, that is, the ability to count out a particular number.
When asked to get a particular quantity:
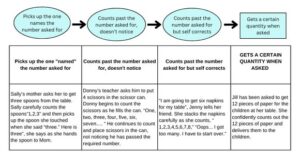
When a child is able to do a task, we are often unaware that the child lacks understanding of the important underlying mathematical concepts. Consider the following example: I observed Josh, a first grade student, filling a container with cubes and counting each cube as he dropped it into the container. He counted accurately as he filled the container with 8 objects. When finished, he wrote the numeral 8 on his paper. He did the task with such ease that I decided to present a more challenging task. Knowing that counting the cubes one at a time and placing them into a container was easier than counting an unorganized pile of cubes, I asked him to fill the container first, then dump out the cubes and see how many he had. This variation of the task would require him to organize and keep track of the objects, something unnecessary when he was counting each one as he put it into the container. So Josh filled a smaller container with 4 cubes, dumped them out as directed and then proceeded to count them. Without the full container as a cue to stop counting, he counted the 4 cubes correctly, but he didn’t stop. He kept on pointing and “counting” them over and over again. I stopped him and asked him to hand me three of the four objects. He handed me one. I then asked him to hand me two objects. He just handed me the rest of the cubes, one at a time. While Josh is older than most children who are at this stage, this level of thinking is not unique or even unusual.
Another child helps us appreciate the complexity of counting. I assessed Cherita by having her count an unorganized pile of 32 objects. She counted those 32 objects correctly, easily keeping track as she went. Then I asked her to make a pile of 18 objects. She began making a pile of counters, but didn’t notice when she got to 18. She kept counting and adding to the pile until she had put all 32 counters into the pile. So I asked her to make a pile of 12. Again she counted until she used all 32 counters. It may appear that the problem was simply a language problem, but I have found that once I am working with a number that has meaning for the child, the instructions are easily followed. This was the case for Cherita. When I asked her to make a pile of 8, she was able to hold that number in mind and stop when she had reached the appropriate amount.
Another critical learning phase that is often overlooked relates to children’s understanding of numbers as tens and ones. Children are often able to label the digits in a number as tens or ones but that does not mean they understand the underlying structure of the numbers.

When children understand numbers are composed of tens and ones, they can learn to combine the tens and ones with relative ease, forming additional tens as needed. However, it is not effective to teach a child to “add the tens” if to them the “tens” are simply digits in a number. They may learn to get answers but without understanding. When children receive instruction before they have the foundational ideas necessary to understand the mathematics present in the problems they are asked to solve, the best they can do is memorize the steps for getting right answers to these problems.
Through my many years of studying children and my research on how they develop mathematical concepts, I know it is essential for teachers to look closely at children’s thinking and recognize the critical learning phases as children move through them if they are going to provide meaningful and effective instruction and ensure maximum learning for each child.
